Slovenian-France "COTUTELLE" Thesis
Author: Andrej Šoštarič
Mentor: Prof. Dr. Damjan Zazula
Co mentor: Prof. Dr. Christian Doncarli
Date: Nov. 28, 1998
Application of wavelet transform and
time-scale representations to the decomposition of compound signals
Keywords: wavelet packets, discriminant bases, feature extraction,
compound signal decomposition, subtractive continuous wavelet analysis,
time-scale phase plane
UDK: 621.391:681.32
Abstract: In the thesis, we
concentrate on application of wavelet based methods on biomedical signals, more
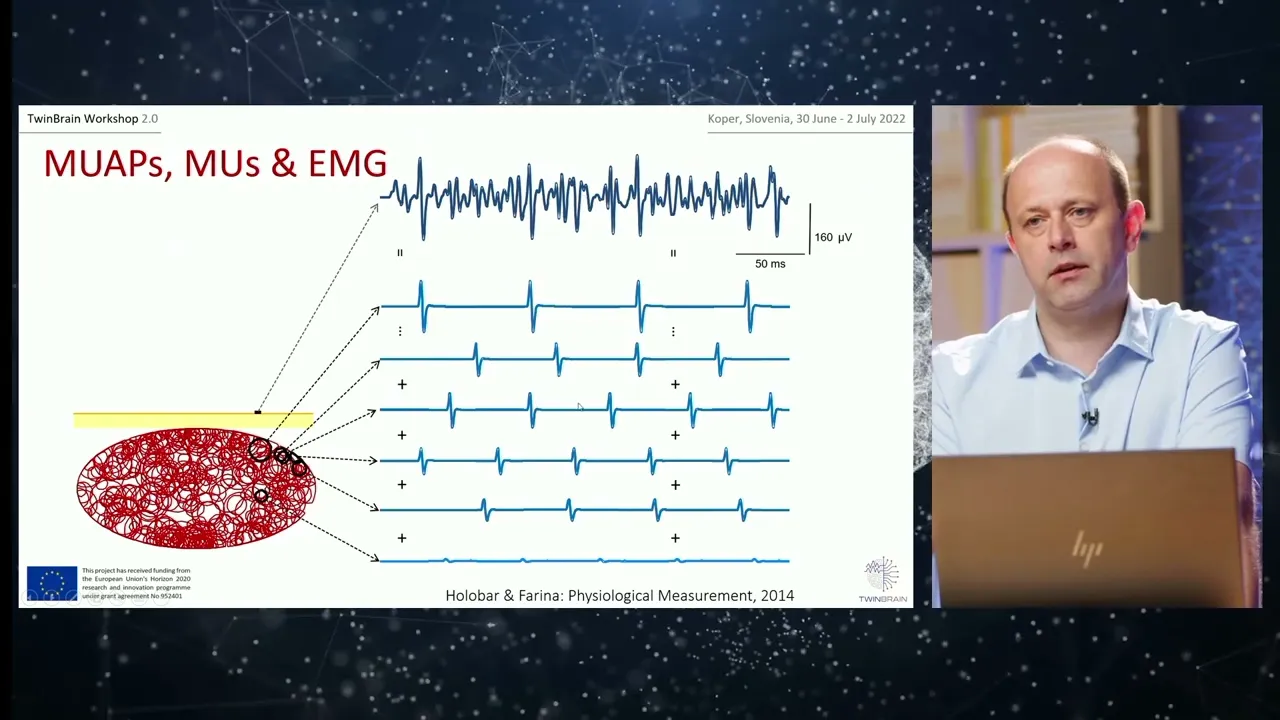
precisely on the EMG signals, because they are representatives of compound
signals. Therefore, the basic physiology and morphology facts (single fiber
action potentials, motor units, etc.) followed by the simplified dipole and
tripole models are being studied. Next, we explore the vast wavelet theory,
starting from the continuous and discrete wavelet transform, multiresolution
analysis, orthogonality of bases, relations between wavelets and filter banks,
and finishing with wavelet packets, best basis algorithm and local discriminant
analysis.
Encouraged by the knowledge obtained from the EMG models, we find a resemblance between the building blocks of the EMGs and the wavelets. Thereafter, we introduce three novel analysis/decomposition techniques: multimodal parametric search (MPS), subtractive wavelet transform (SWT) and time-scale phase decomposition. MPS and SWT techniques are based on searching the best fit action potential in an optimal way, the former considering the error generated by the Euclidean distance metrics, while the latter using the cross-correlation. Two-parameter plane obtained by the MPS and time-scale plane by the SWT contain equivalent information. Even though they both suffer from the same disadvantages, they can be used for the analysis of the EMG signals at lower contraction forces, when the number of superimposed components is reasonably low.
We obtain more precise information about the
building blocks superimposed in the (synthetic) EMG signals with the time-scale
phase
analysis. To obtain time-scale phase plane (TSPP) we use the implicit phase
defined upon the half-open interval [0, 2\pi) as four quadrant arctangent
function instead of using a standard arctangent function, which represents a
unique approach in time-scale analysis. Applying the time-scale phase analysis
to the synthetic EMG signals, we have shown that this technique is superior to
the MPS and SWT in detection of correct firing positions of building blocks in
time. Although the technique is very sensitive to noise, we think that the use
of implicit phase in time-scale analysis is a very interesting one and is worth
of further investigation.
Beside the novel methods, we have also successfully applied some adapted wavelet and wavelet packets based analysis techniques to filtering, smoothing and feature extracion of real and synthetic EMG signals.